· Pattern

· Understands the concepts of “even numbers” and “odd numbers”
· Can count by 2’s, 5’s and 10’s
· Can identify and describe patterns in a collection of related numerical data

· four crayons or markers of different colors (each student)

Procedure: This activity is best done by students working individually.

On the day of this lesson, wear an article of clothing that has a clear, brightly colored pattern. Up until the point that the lesson begins, have some music playing. Turn off the music and start the lesson by asking students to notice this clothing, and ask what they observe about it.

Elicit the response that the clothing has a pattern to it. Lead this into discussion of patterns and definition of “pattern” (a rule or plan that tells how something is made).

Then say, “You noticed there is a pattern on my clothing, but did you notice that there was another pattern in the room when we started class? Can anyone guess what that was?”

Lead students to the realization that there is a pattern to the music.

Ask students to give more examples of patterns. For number or shape patterns, write those on the board. Be sure in each case to discuss the rule or plan behind the pattern.

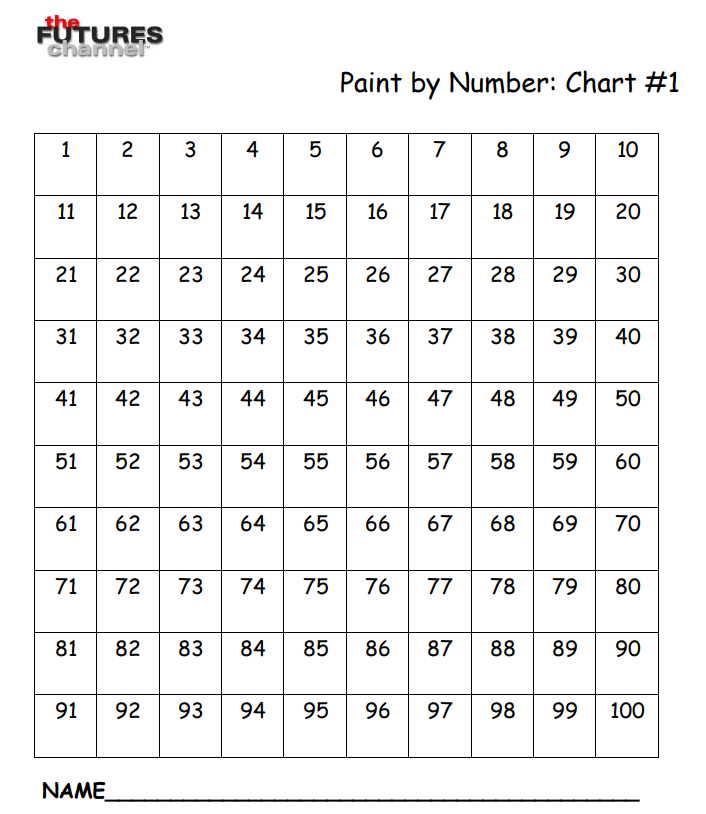
Tell the students you have more patterns for them to look at, and distribute handout #1. (If possible, have a large version of the chart in the front of the room as well, copied on the blackboard or by using an overhead projector). Ask students to tell you what patterns they observe in this chart. For each pattern, ask them to explain what the rule or plan is. Be sure to discuss the patterns which involve odd and even numbers (any column contains only odd or only even numbers), and patterns of multiples (the column starting with “5” or “10”, the diagonal that starts with “11”, the diagonal that starts with “9”).

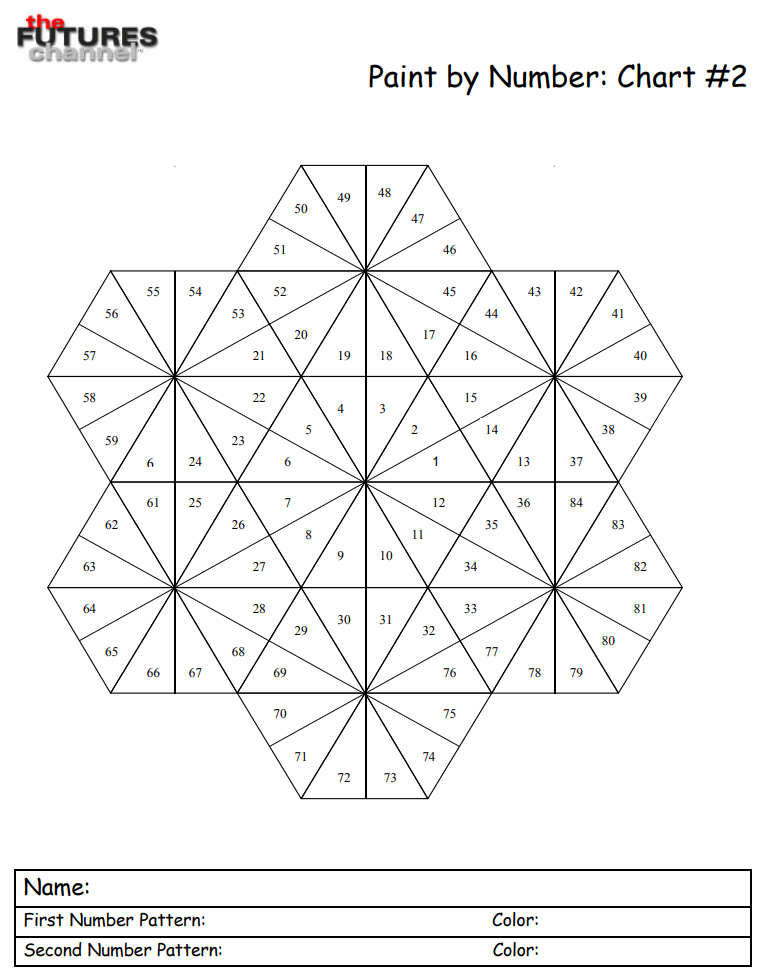
Repeat this process with chart #2 (this time students will probably come up with geometrical patterns that they see in the design).

Then tell students that you have a project for them, as follows:

1) Students will make up a number pattern, or they can choose one of those discussed earlier from the blackboard. (Examples: 2,4,6,8…; 3,6,9,12,…; the Fibonacci sequence—1,2,3,5,8,13…; etc.)

2) They write down the number pattern on the bottom of the #2 handout.

3) They will color in all of the numbers from that number pattern on chart #2, using one color.

4) Repeat steps 1 to 3 with a second number pattern and a different color.

5) Color in all remaining spaces on chart #2 with a third color.

This activity should take twenty to thirty minutes. While students are working on it, circulate and ensure they are following directions by asking them questions about what they are doing.

When most or all students have finished, stop the activity and have some of the students show their results and explain their number patterns. (You may wish to put all of the students’ patterns on a bulletin board.)

Wrap up with a review of the definition of pattern.